E=mc², a simple demo

(last edited on 4th June 2020)

E = mc² (E = energy, m= mass, c = speed of light) is the most well-known of all equations in physics and yet its origin and meaning are seldom explained in a simple way . The equation arose out of Einstein’s Theory of (Special) Relativity, and, according to Einstein, is the most important outcome of that theory.

To explain E = mc² we present a thought-experiment of Einstein’s, as made simple by Hermann Bondi in the latter’s book, “Relativity and Common Sense”.

Consider a stationary box that is isolated (external influences, such as gravity, friction, etc., are assumed small enough to be ignorable). As the box is not moving, we know that its momentum is zero (momentum = mass x speed in a given direction). But then something happens…

The isolated box, having been stationary, now starts to move! From the cherished principle of the conservation of momentum, we know that the momentum must still be zero (there have been no external influences). The only way this can be so is if, while the box goes in one direction, an internal mass goes in the opposite direction. (However many masses are inside the box, we can always consider their net effect as being equivalent to just one internal mass.) The process can be split into three stages, as follows (for simplicity, we’ll assume directions ‘left’ and ‘right’):

(1) at the left end of the box there is a ‘gun’ on hold; (2) an internal mechanism, with timer, fires the gun and this makes a ‘bullet-mass’ travel to the right and the box recoil to the left; (3) the ‘bullet-mass’ collides with the approaching right-hand end of the box and the motions of both bullet and box are brought to zero; also, the bullet gets stuck in the flypaper and its kinetic energy is dissipated into making this flypaper get squashed and then hot. (To enlarge, hold cursor over diagram and click)

So, all we see is that there is a box that is still, then it starts moving, and then it stops (in a new location). We have said nothing about how big the box is, or how fast it travels – but the conservation of momentum must hold true whatever the values (tiny masses, huge masses, high speeds, low speeds, a long box, a short box).

Einstein’s brilliant thought-experiment was to suppose that, instead of a gun that fires a mass, we have a gun that fires a pulse of light. This new gun (flash gun) is also triggered by an internal timer, but has a mirror at the flash-bulb end and black paint at the far end of the box. The recoil of the box is now tiny (when we take a photo and use the flash, we don’t get punched backwards by the camera!) but is still there, in principle. The speed of the pulse is very fast (the speed of light, of course) and, instead of sticky tape, the pulse is absorbed by and warms up the black coating at the right-hand end of the box:

(To enlarge, hold cursor over diagram and click)

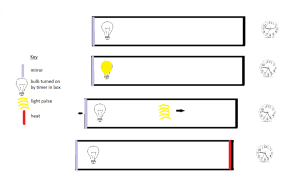
We can’t see inside the box and so the two scenarios (bullet-in-box or flash-in-box) appear identical (apart from questions of scale). Therefore, in the case of the flash-in-box, once again, the conservation of momentum must apply, and, once again, there must be an internal rearrangement of mass from the left to the right ends of the box. However, all we find when we open the box (after its recoil motion has stopped) is that its inner right-hand end is hot. Therefore, we are forced to conclude that heat has mass.

As extra confirmation that the argument is correct, there is much evidence, independent of Special Relativity, that light has momentum. For example, it can be shown that light falling on a thin metal vane suspended in a vacuum will push the vane sideways (the Nichols’s radiometer).

You have learned the most important part of Special Relativity – that energy and mass are equivalent to each other, E = m. We could stop here, and many physicists do, in fact, choose units such that c=1, but if you want to find out where E = mc² comes from the derivation is given near the end of this article. A curious irony is that pure light has no mass (no rest mass) – further discussion at the end of the article.

Einstein quickly realized that this equation was to apply to any kind of energy. Any kind of energy, whether the energy of motion, of heat, light, electricity, chemical action, etc. has a mass, and this mass has the usual dynamic implications (it has ‘inertia’) and the usual gravitational implications (it attracts and is attracted by other masses). Arguing the other way, Einstein also realized that any kind of mass (whether stationary, moving, radioactive, non-radioactive, etc.) implies an equivalent amount of energy. Whether this energy could actually be tapped, e.g. whether the energy associated with, say, a proton mass could ever be released, was a matter for experiment to determine. It has since been found, for example in high-energy accelerator laboratories, that mass can be converted into energy. We also understand that our Sun works in this way.

We are familiar with the idea that the Theory of Special Relativity explains what happens when particles travel at very high speeds, close to the speed of light. However, it is not only in this high-speed regime that the Theory is important: the consequences of Special Relativity are far-reaching and increase our understanding of all physics. For example, E = mc² applies to slow-moving or even stationary masses.

The Experiment

You may be wondering how I made the box move. First of all a battery-powered gun was tried but the batteries and the metal slug inside the solenoid were all too heavy (making the recoil of the box too feeble). Then a mousetrap release mechanism was tried with a little funnel to drop sand onto the trigger: but the mechanism smashed the funnel, fingers were hurt, and the floor soon became littered with sand, wheat grains etc. Finally, a mechanical fly-zapper was tried with the trigger modified to incorporate a gingernut biscuit (too soft) and then a sugar cube dosed with just the right amount of water – SUCCESS! All of which just goes to highlight two other important principles in physics: (1) nature abhors a discontinuity (for example, an in-built time-delay), (2) it is difficult to carry out a thought-experiment in practice.

—————————————————————————————–

Derivation of E = mc²

We imagine a box of length, L, with the centre of mass at L/2. The mass of the box is m_b and the transferred mass is m_tfr. The displacement of the box, along its length, (after the light-flash) is ΔS.

From conservation of momentum we know that:

momentum of box = momentum of flash = p (1)

Also:

momentum of box = m_b* speed of box = m_b * ΔS * 1/Δt = p (2)

Now Δt = time-of-flight of light flash = L/c (3)

Therefore ΔS = p Δt/m_b = pL/(cm_b) . Crucial to the derivation is that, from Maxwell’s Theory of Light, it can be shown that the momentum of light is equal to its energy divided by the speed c,

p = E/c (4)

However we remember that Maxwell’s Theory arrived before the Theory of Special Relativity.

From (1) to (4) we find:

ΔS = EL/(c²m_b) (5)

The centre of mass doesn’t move (this follows from the conservation of total momentum). Let’s take `moments’ about one end of the box,

total moment before box moves = total moment after box has moved

m_tfr* zero + m_b* L/2 = m_tfr * (L – ΔS) + m_b* (L/2 – ΔS) (6)

Now as ΔS m_tfr ≈ 0 (both ΔS and m_tfrare very small) we end up with Lm_tfr = ΔS m_b and so

ΔS = L m_tfr/ m_b (7)

Equating (5) and (7), and cancelling L/m_b, we find

m_tfr =E/ c² (8)

Rearranging, we finally obtain

E = m_tfr c²

Mass of Light?

It is curious to note that we know that the photon – the light particle – has no mass (that is to say, it has no ‘rest mass’.) However, there are many scenarios where light does have a ‘mass equivalent’. For example, the Sun loses mass year by year, and this mass is chiefly irradiated away, it is lost as light (some mass is also lost in coronal mass ejections, solar wind, etc.). Also, if a particle approaches its antiparticle and they both annihilate then two gamma rays will be produced. The masses of the particle and the antiparticle can be added. This mass-sum must still be present after the annihilation (e.g. suppose it all happens in a perfectly closed box – it will make no difference to us whether we view the box before, during, or after the annihilation). The trick is to understand that all these scenarios (light-gun in a box, the Sun, annihilation) involve a combination of light and matter, none involve pure light. Or if you want to be quantum mechanical philosophical about it, you could say “whenever we actually perform an experiment, then there’s always some mass around, it’s never just pure light”.

—————————————————————————————-

More philosophy

One of the biggest insights to come out of the equivalence of energy and mass, is to resolve a curious feature of classical mechanics. In classical mechanics, the kinetic energy of a body can become zero depending on which reference frame it is viewed from; and a body that is stationary can acquire kinetic energy depending on which reference frame it is viewed from. In Special Relativity this puzzle is resolved: because a mass always has a finite (non-zero) and positive energy, whether the mass is moving or not, then the following is now true

Any system which has zero energy will always have zero energy, howsoever the system is viewed,

and

Any system which has finite energy will always have finite energy, howsoever the system is viewed.

(Terminology: ‘viewer’ is the same thing as ‘valid reference frame’, and ‘system’ is a shorthand for ‘isolated system’.)

It seems that the energy of a system has the property of being a sort of tally – there’s something there or there’s nothing there… This existential property must surely be true in all domains of physics (including general relativity and cosmology), but I have not seen this demonstrated.

Note that the same cannot be said of the total momentum of a system: a finite momentum can become zero; a zero momentum can become finite – depending on how the system is viewed. Note also that the actual amount of finite energy, 12 Joules, 51112.9 Joules, or whatever, will not in general be the same for different viewers. In other words, energy is not an ‘invariant’ quantity even while it is conserved in any given isolated system.

Share this: